INTERVALS
-
Interval: the distance in pitch between two notes
Melodic Interval: the distance between two notes sounding successively
Harmonic Interval: the distance between two notes sounding simultaneously
Any interval name is divided in two parts: a number and a modifier preceeding the number.
The numerical part of an interval is an integer number: 1, 2, 3, ......n; these numbers are referred to as thirds (or 3rds), fifths (or 5ths), and so on with the exception of the 1 and the 8 instead of which the terms unison and octave (or 8ve) are used. Intervals form the unison to the octave are called simple intervals ; all the others are called compound intervals and they are formed by some combination of an x number of octaves plus a simple interval, which makes them behave as their simple component.
The numbering system is based upon the degrees of the major scale and that is why even though there are 13 notes in an octave we only use 8 numbers. Each degree or note of the scale corresponds to a specific number which means, for example, that the interval C-G is only and always a fifth regardless of alterations. At this point, since the notes can be altered in both directions by means of flats and sharps, we need something that takes these variables into account so that we will know precisely the distance between the two notes. Here is where the modifiers become essential. Their function is to label the direction of the alteration (if any) so that we can precisely identify any interval within the octave. The modifiers' names are:
The meaning of these names is derived from the relationship between the notes (frequency ratio) in the harmonic series.
The modifiers are also based on the major scale. In Ex.1 you can see how every interval between any note in the C major scale and its tonic is either perfect or major.
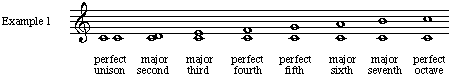
As you can see the term perfect
is used with the unison, the 4th, the 5th, and the 8ve while major is used with all the others.
In order to determine the exact distance between two notes in a given interval we need to know how many half steps there are between them:
The fact that the interval number and the corresponding number of half steps do not match (with the exception of the 2nd) might seem a little confusing at first, but remember that there is no relation between the two whatsoever.
This nomenclature is one out of the many existing ways to catalog the chromatic scale. One of these, for example, identifies intervals by numbering them accordingly to the number of half steps between the notes. In the latter system a 7th would be what we call a perfect fifth. The reasons why we study this system instead of another are 1) because it is the most widely used and 2) because of tonality; since 99% of the music we deal with every day is mostly tonal this nomenclature is the one that most efficiently classifies it.
Now that we know exaclty what these intervals mean let's take a look at the remaining modifiers.
-
Minor Interval: a major interval made smaller by a 1/2 step without altering its numerical name
Augmented Interval: a perfect or major interval made larger by a 1/2 step without altering its numerical name
Diminished Interval: a perfect or minor interval made smaller by a 1/2 step without altering its numerical name
Double Diminished: a diminished interval made smaller by a 1/2 step without altering its numerical name
Double Augmented: an augmented interval made larger by a 1/2 step without altering its numerical name
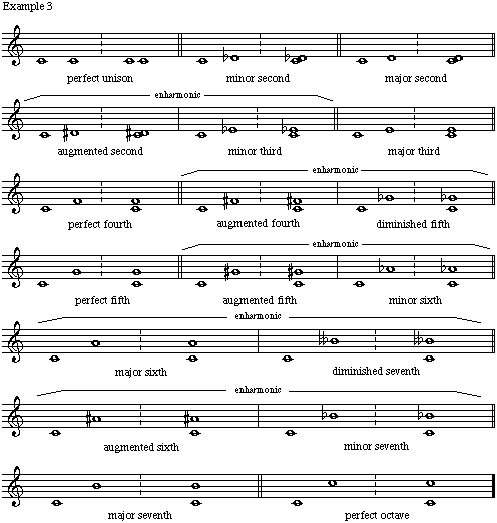
It is imperative for the numerical name to remain constant when we alter an interval otherwise we will end up with a different one! For example, let's say we have the same interval C-G and this time we specify that there are no alterations so that the interval is a perfect fifth; if we lower the G by a 1/2 step we obtain a new interval C-Gb which is a "diminished fifth." Now, if we raise the C by a half step we will have C#-Gb as our new interval and since the numerical name is still a 5 (for 5th) we must call it a "double diminished fifth." Suppose that we want to count the half steps between the two notes, we would find out that there are 5 of them: C -(1)- D -(2)- D# -(3)- E -(4)- F -(5)- Gb. But this is the number of half steps for a "perfect fourth"! Yes, also. These kind of intervals are called enharmonic : two different interval names used for the same "distance in pitch." This can be confusing and you might wonder why can't we just use one name for each pitch distance. The answer is that most of the time we do, it is much more common to encounter a perfect fourth than a double diminished fifth since, on tempered instruments, they sound exactly the same. But sometimes another name might be more appropriate; instruments that are not tempered, for example, will play a double diminished fifth a little sharper that a perfect fourth.
As we have seen before the term "perfect" is only used in connection with unisons, fourths, fifths, and octaves, while seconds, thirds, and sixths can only be major or, as we know now, minor. Augmented and diminished, and obviously their doubles apply indifferently to all of them. You can also have triple and quadruple augmentations and diminutions even though they are rarely encountered, but at least it should be clear that after the doubling the behaviour of the interval remains constant.
Every rule about the modifiers can be reversed; for example, minor intervals made larger by a 1/2 step without altering their numerical name become major intervals. But while this is always true for the major and minor modifiers we have to be careful with the diminished and the agumented ones. As we have seen the augmented and diminished modifiers can apply to every numerical interval; for this reason if we enlarge a diminished interval by a 1/2 step the resulting interval will depend on the numerical name: a perfect interval if it was a unison, a fourth, a fifth or an octave, or a minor interval if it was a second, a third, or a sixth.
One last note before taking a look at the inversions: the term tritone is sometimes used instead of the augmented 4th. This is because of the three whole steps which characterize this particular interval.
The following chart lists the most common simple intervals.
Compound intervals, as we said, have the same behaviour of their simple components; the only difference is in their numerical number. In order to isolate the simple interval in a compound one we subtract the number "7" from the numerical number of the latter until we obtain a number between 1 and 8. That number is the numerical of the simple component. For example, a 13th behaves like a sixth (13-7=6). The number "7" is used instead of "8" because we number the degrees of the scale from 1 to 8 thus omitting the zero.
INVERSION OF INTERVALS
Just as every particle of matter in the universe has its own anti-material counterpart, every interval has its own unique inversion.
An interval is inverted by transposing the bottom note up by an octave or vice versa. For example, the inversion of the interval C-G is G-C. Note that for compound intervals you must also add the number of octaves that are in the interval itself multiplied by two.
When an interval is inverted the numerical name and the modifier change accordingly with the inversion. The new numerical name can be calculated by subtracting the old one from the number "9" (same reason as for the number "7" for the compound intervals). The modifiers change as follow:
-
Major intervals become minor intervals
Minor intervals become major intervals
Perfect intervals remain perfect intervals
Augmented intervals become diminished intervals
Diminished intervals become augmented intervals
Again, double and triple augmented or diminished intervals behave as the augmented and diminished ones.
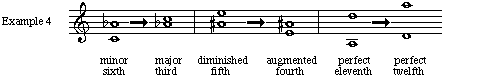
A good understanding of intervals is the base for the understanding of scales, chords, harmony, and every other musical aspect concerning pitches.
Sometimes we tend to dismiss as superfluous a particular theoretical aspect of music just because we cannot see their practical implication. Until we need it........
